• Гідрологія і Гідрометрія
• Господарське право
• Економіка будівництва
• Економіка природокористування
• Економічна теорія
• Земельне право
• Історія України
• Кримінально виконавче право
• Медична радіологія
• Методи аналізу
• Міжнародне приватне право
• Міжнародний маркетинг
• Основи екології
• Предмет Політологія
• Соціальне страхування
• Технічні засоби організації дорожнього руху
• Товарознавство продовольчих товарів

Rotary Motions

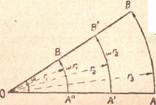
Angular Velocity. — Let one end of a line ОA of Figure 18 be fixed, and then let the line revolve in a plane about this fixed end. The rate at which the line rotates is called its angular velocity which is usually expressed in radians per second. If the rate of rotation of the line is constant, the angular velocity is constant and is equal to the angle through which the line turns in unit time. The angular velocity may also be measured in revolutions per second or per minute. Angu­lar velocity like linear velocity is a vector quantity. It can be repre­sented by drawing a line of suitable length in the direction of the axis about which the rotation takes place.

Angular Acceleration. — The rate at which the angular velocity changes is called the angular acceleration. It is the increase or de­crease in angular velocity per unit of time. It is related to the angular velocity in the same way in which the linear acceleration is relat­ed to linear velocity. In an angular acceleration as in linear accelera­tion it is necessary to specify two units of time. One of these units gives the unit of time in which the angular velocity is measured, and the other gives the unit of time used to measure the change in the angular velocity. Ordinarily, the same unit of time is used in both cases.

Переглядів: 473